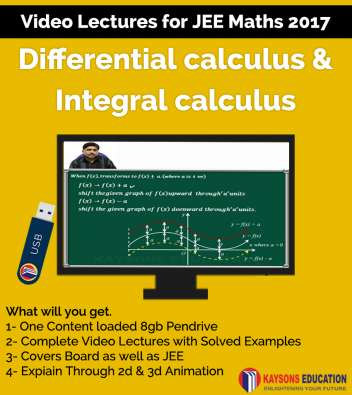
Day 1: Fundamentals, Basic Definitions, CLOSED AND OPEN INTERVALS, Modulus or Absolute Value Function, Generalised Results, Wavy Curve Method/Number Line Rule/ Sign Scheme For Rational Function, Fundamentals Of Quadratic Equations, The sum of several non-negative terms.
Day 2: Definition, Independent and dependent variable, Graphical representation of function, Real Function, Content function, Identity function, Modulus function, Properties of modulus function, The greatest integer function, Properties of greatest integral function, Fractional part function, Properties of fractional part of x, Least integer function, Properties of least integer function, Signum Function, Reciprocal function, Logarithmic function, Exponential function, Square root function, Polynomial function, Rational function, Inverse of trigonometric function, Operations on real function, Composition of functions, Rule for Finding Domain.
Day 3: Monotonic Function, Nature of derivative of function, Nature of derivative, Range, Method to find the range of a function y = f(x), Odd and even function, Odd function, Even Function, Properties of odd and even function, Periodic function, Properties of periodic function, While taking LCM we should always remember.
Day 4: Mapping of Function, Kinds of function, One-one Mapping or injective or monomorphic, Method to check one-one mapping, Number of one – one Mapping, Method to check Many-One, Onto Function or (Subjective), Method of show onto or subjective, Number of onto Functions, Number of one – one onto mapping or bijection, Equal and identical function, Inverse of function, Graph of the inverse of an invertible function, Properties of inverse of a function, Composite functions, The adjacent figure shows the steps to be taken, Properties of Composition of function.
Day 5: Illustration.
Day 6: Illustration.
Limit
Day 1: Basic concept, Fundamental algebraic operation on limits of function, Standard limits, Indeterminate forms, Sandwich theorem, Some important expansions, Factorization method, Rationalization Method, Based on standard formula.
Day 2: Algebraic function of ∞ type ∞/∞ form, How to solve problems, Trigonometrical Limits, Logarithmic Limits, Exponential Limits.
Day 3: Based on definition of ‘e’, Evaluation of exponential limits of the form 1∞, Particular cases, Miscellaneous Forms, Use of Sandwich theorem (Squeeze theorem), Use of Newton-Leibnitz’s formula in evaluating the limits, L’ Hospital’s Theorem, Advance level.
Day 4: Illustration.
Continuity and Differentiability
Day 1: Continuity of a function, Graphical View, Continuity at end points, Jump discontinuity, Properties of continuity function, Differentiability.
Day 2: Differentiability in a set, Some standard results on differentiability.
Day 3: Illustration.
DVD 2
Differentiation
Day 1: Introduction.
Day 2: Derivative of standards functions, Rule (i), Rule (ii) ( Product Rule), Generalization of the product rule, Rule (iii) (Quotient rule), Differentiation of a function of a function, Differentiation by using trigonometrical transformations.
Day 3: Differentiation of implicit functions, Logarithmic Differentiation, Differentiation of parametric functions, Differentiation of a function with respect to another function, Differentiation of determinants.
Day 4: Illustration.
Application and Derivatives
Day 1: Derivative as a rate measurer, Derivative as the time rate of change, Differentials, Errors and Approximations, Rolle’s & Lagrange’s Theorem, Rolle’s Theorem, Geometrical proof, Lagrange’s Theorem, Geometrical interpretation.
Day 2: Illustration
Tangents and Normals
Day 1: Basic definition, Slope (Gradient) of a line, Slope of a line in terms of coordinates of any two points on it, Slope of a line when its equation is given, Angle between two lines, Equation of a straight line.
Day 2: Illustration.
Monotonicity
Day 1: Monotonic function.
Day 2: Properties of Monotonic Functions, Advance level Include Subjective type questions.
Day 3: Illustration.
Maxima Minima
Day 1: Definition, Maxima and Minima at end point, Method of finding extrema of continuous functions, Method of 2nd derivative, Concept of Global Maximum / Minimum, Global Maximum / Minimum in [a, b], Global Maximum / Minimum in (a, b).
Day 2: Applied problems in maxima and minima.
Day 3: Maxima and Minima in Discontinuous Function, Minimum of discontinuous functions, Maximum of discontinuous functions, Method of finding the greatest and least values of a continuous function.
Day 4: Illustration.
Graphical Transformation
Day 1: Some Standard Graphs, Straight Line, Ellipse, Hyperbola, Rectangular Hyperbola, Transformation of Graphs, How to draw graph of Polynomial, Draw the graph, Plotting graph of f(x – [x]),